The Exponential Function

A LevelAQAEdexcelOCR

The Exponential Function Revision

The Exponential Function

We have met exponential functions before, but there is one specific exponential function that has special properties, and it is based around a special number: $\color{red}e$ .

e

The exponential function is $\color{red}e^{x}$ .

$\color{red}e=2.71828...$ is a number. It is a decimal that goes on forever
(like $\pi$ ).

$\color{red}e^{x}$ has special properties, most notable being that the gradient of $\color{red}e^{x}$ is $\color{red}e^{x}$ . This will be very important in the differentiation section of the course.

There are some key facts to remember about the graph of $y=e^{x}$ :

It crosses the $y$ -axis at $(0,1)$
As $x\rightarrow\infty$ , $\color{red}e^{x}\color{grey}\rightarrow\infty$ and as $x\rightarrow -\infty$ , $\color{red}e^{x}\color{grey}\rightarrow0$
$\color{red}e^{x}$ is never negative.

$y=e^{ax+b} + c$ is a transformation of $y = e^x$ , where $a$ is a horizontal stretch, $b$ moves it horizontally and $c$ moves it vertically.

$y = e^{-x}$ reflects $y=e^x$ in the $y$ -axis.

Natural Logarithm

The inverse function of $\color{red}e^{x}$ is the natural logarithm $\color{blue}\ln(x)$ . This is the logarithm with base $\color{red}e$ $(\text{log}_e (x))$ .

All the laws of logarithms can be applied to the natural logarithm.

$\color{blue}\ln(a)\color{grey}+\color{blue}\ln(b)\color{grey}=\color{blue}\ln(ab)$

$\color{blue}\ln(a)\color{grey}-\color{blue}\ln(b)\color{grey}=\color{blue}\ln\left(\dfrac{a}{b}\right)$

$\color{blue}\ln(a^{b})\color{grey}=\color{blue}b\ln(a)$

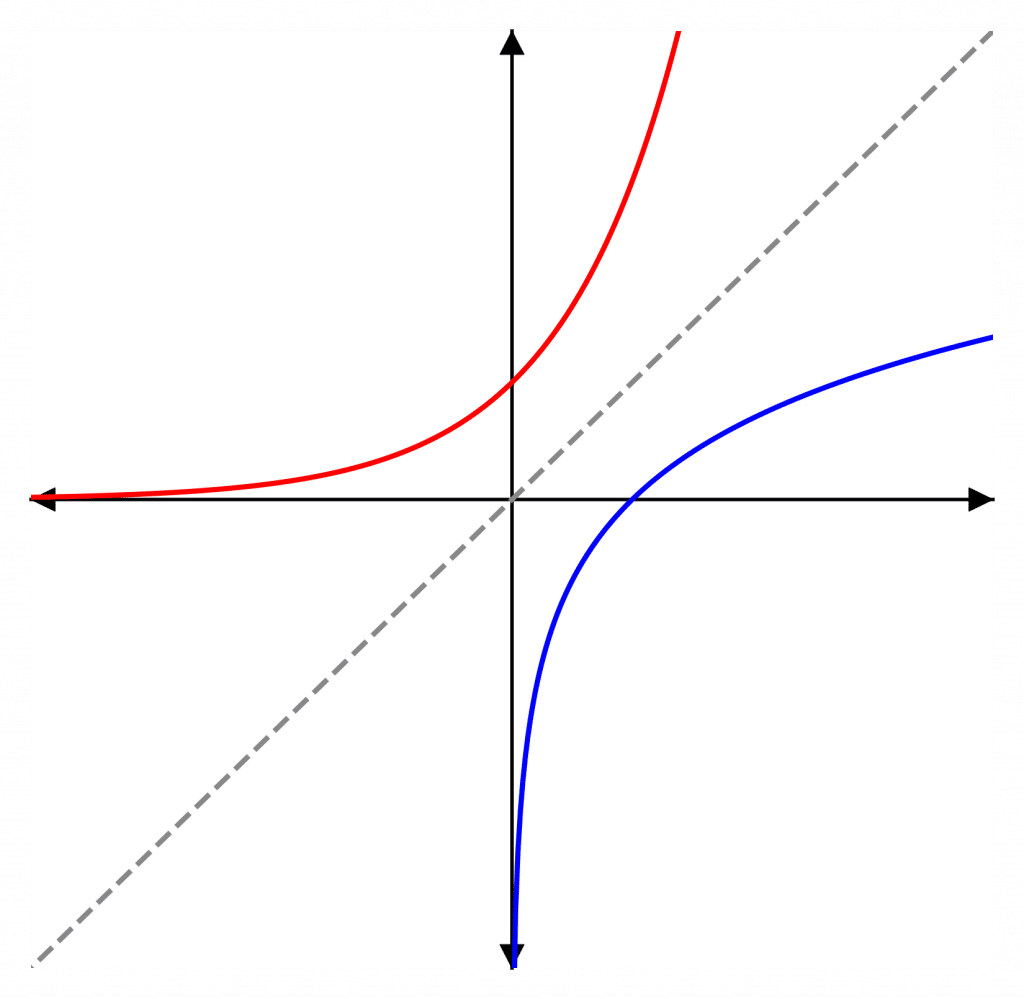
The graph of the natural logarithm (in blue) is the reflection in the line $y=x$ of the graph of the exponential function (in red).

There are key facts to remember about the graph of $\color{blue}y=\ln(x)$ :

It crosses the $x$ -axis at $(1,0)$
As $x\rightarrow\infty$ , $\color{blue}\ln(x)\color{grey}\rightarrow\infty$ and as $x\rightarrow0$ , $\color{blue}\ln(x)\color{grey}\rightarrow -\infty$
$\color{blue}\ln(x)$ does not take any values for $x\leq0$

Since $\ln (x)$ is the inverse of $e^x$ and is a logarithmic function, we have these formulas relating the two:

$\textcolor{red}{e}^{\textcolor{blue}{\ln (x)}} = x$

$\textcolor{blue}{\ln} \textcolor{red}{(e^x)} = x$

Example 1: Equations Involving the Exponential Function

Solve for $x$ :

\color{red}e^{3x}\color{grey}=10

[2 marks]

$\color{red}e^{3x}\color{grey}=10$

$3x=\color{blue}\ln(10)$

$\begin{aligned}x&=\dfrac{\color{blue}{\ln(10)}}{3}\\[1.2em]&=0.768\end{aligned}$

Example 2: Equations Involving Logarithms

Solve for $x$ :

\color{blue}\ln(4x+3)\color{grey}=2

[2 marks]

$\color{blue}\ln(4x+3)\color{grey}=2$

$4x+3=\color{red}{e^{2}}$

$4x=\color{red}e^{2}\color{grey}-3$

$\begin{aligned}x&=\dfrac{1}{4}(\color{red}e^{2}\color{grey}-3)\\[1.2em]&=1.10\end{aligned}$

The Exponential Function Example Questions

Question 1: Solve for $x$ :

a) $e^{x}=2$

a) $e^{5x}=19$

c) $e^{12x}=234$

[6 marks]

a) $e^{x}=2$

$\begin{aligned}x&=\ln(2)\\[1.2em]&=0.693\end{aligned}$

b) $e^{5x}=19$

$5x=\ln(19)$

$\begin{aligned}x&=\dfrac{1}{5}\ln(19)\\[1.2em]&=0.589\end{aligned}$

c) $e^{12x}=234$

$12x=\ln(234)$

$\begin{aligned}x&=\dfrac{1}{12}\ln(234)\\[1.2em]&=0.455\end{aligned}$

Save your answers with

Gold Standard Education

Question 2: Solve for $x$ :

a) $\ln(x+1)=4$

a) $\ln(3x+2)=1.5$

a) $\ln(9x+36)=0.6$

[6 marks]

a) $\ln(x+1)=4$

$x+1=e^{4}$

$\begin{aligned}x&=e^{4}-1\\[1.2em]&=53.6\end{aligned}$

b) $\ln(3x+2)=1.5$

$3x+2=e^{1.5}$

$3x=e^{1.5}-2$

$\begin{aligned}x&=\dfrac{1}{3}(e^{1.5}-2)\\[1.2em]&=0.827\end{aligned}$

c) $\ln(9x+36)=0.6$

$9x+36=e^{0.6}$

$9x=e^{0.6}-36$

$\begin{aligned}x&=\dfrac{1}{9}(e^{0.6}-36)\\[1.2em]&=-3.80\end{aligned}$

Save your answers with

Gold Standard Education

Question 3: Solve for $x$ :

e^{2x}-13e^{x}+36=0

[4 marks]

e^{2x}-13e^{x}+36=0

Note that $e^{2x}=(e^{x})^{2}$

(e^{x})^{2}-13e^{x}+36=0

Substitute: $y=e^{x}$

$y^{2}-13y+36=0$

$(y-9)(y-4)=0$

$y=9$ or $y=4$

Reverse substitution:

$e^{x}=9$ or $e^{x}=4$

$x=\ln(9)$ or $x=\ln(4)$

$x=2.20$ or $x=1.39$

Save your answers with

Gold Standard Education

Question 4: Solve for $x$ :

\ln(4x+3)-2\ln(x)=5

[5 marks]

$\ln(4x+3)-2\ln(x)=5$

$\ln(4x+3)-\ln(x^{2})=5$

$\ln\left(\dfrac{4x+3}{x^{2}}\right)=5$

$\dfrac{4x+3}{x^{2}}=e^{5}$

$4x+3=e^{5}x^{2}$

$e^{5}x^{2}-4x-3=0$

Use quadratic formula:

$\begin{aligned}x&=\dfrac{-b\pm\sqrt{b^{2}-4ac}}{2a}\\[1.2em]&=\dfrac{4\pm\sqrt{(-4)^{2}-4\times e^{5}\times(-3)}}{2e^{5}}\\[1.2em]&=\dfrac{4\pm\sqrt{16+12e^{5}}}{2e^{5}}\\[1.2em]&=\dfrac{2\pm\sqrt{4+3e^{5}}}{e^{5}}\end{aligned}$

$x=0.156$ or $x=-0.129$

We can discount the negative solution because $\ln(x)$ is not valid for negative $x$ .