Circle Graphs and Tangents

GCSELevel 6-7Level 8-9AQAEdexcelOCR

Circle Graphs and Tangents Revision

Circle Graphs and Tangents

Circle graphs are another type of graph you need to know about. Questions involving circle graphs are some of the hardest on the course.

You need to be able to plot them as well as calculate the equation of tangents to them.

Make sure you are happy with the following topics

What are Circle Graphs?

Circle graphs for a circle, have the general equation

$\textcolor{red}{x}^2 + \textcolor{limegreen}{y}^2 = \textcolor{blue}{r}^2$

e.g:

$\textcolor{red}{x}^2 + \textcolor{limegreen}{y}^2 = \textcolor{blue}{4}^2$

which is the same as

$\textcolor{red}{x}^2 + \textcolor{limegreen}{y}^2 = \textcolor{blue}{16}$

Remember: $r$ is the radius of the circle formed around centre $(0,0)$

Finding the Equation of a Tangent

This is the most typical exam question you will face, if you learn the steps it really isn’t as tricky as it seems.

Example: Find the equation of the tangent to the circle defined by

$x^2 + y^2 = \textcolor{red}{25}$

at the point $(3, 4)$ , shown on the axes below.

Step 1: Find the gradient of the radius.

Firstly, we can recognise that because $\textcolor{red}{5^2} = \textcolor{red}{25}$ , the radius of this circle is $\textcolor{red}{5}$ .

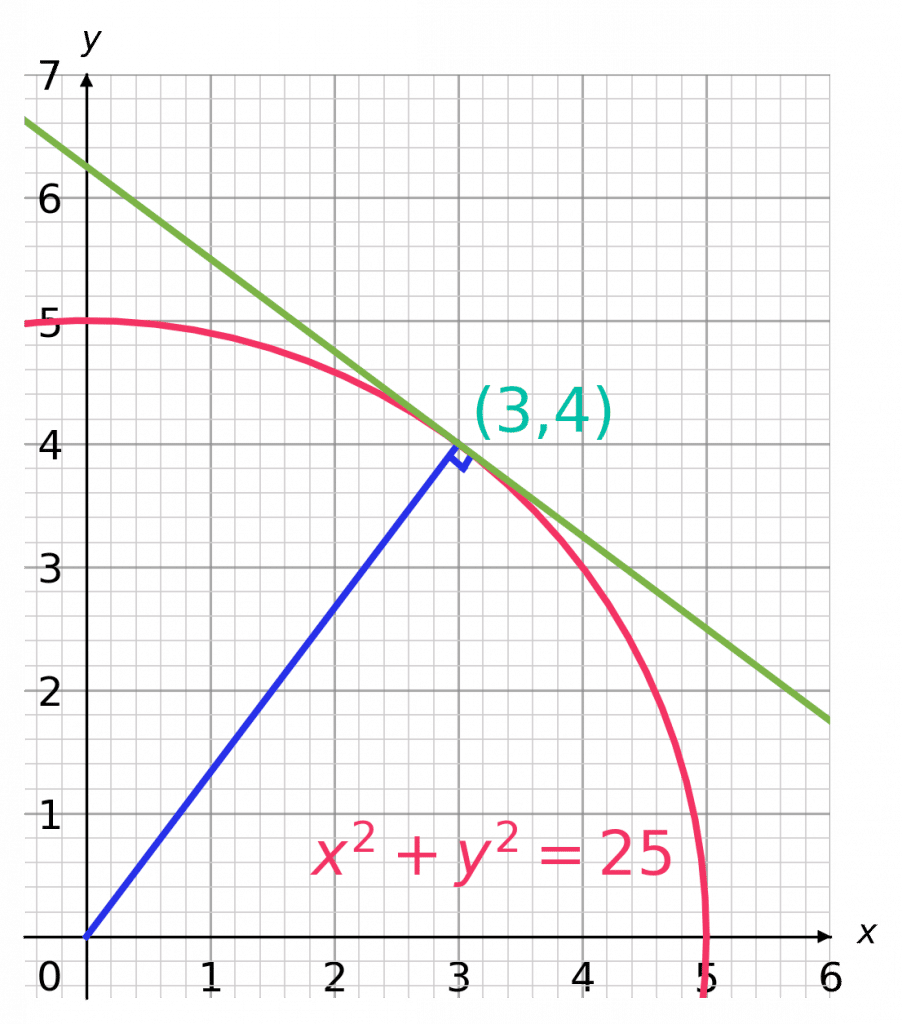
We need to find the gradient of the radius which goes from the centre of the circle to the point $(3,4)$ .

The tangent is perpendicular to the radius at that point (one of our circle theorems), meaning you can obtain the gradient of the perpendicular by taking the negative reciprocal of it.

So, the gradient of the line that goes from the origin to $(3,4)$ is

$\begin{aligned} \text{Gradient } &= \dfrac{\text{change in } y}{\text{change in }x} \\ &= \dfrac{4 - 0}{3 - 0} \\ &= \dfrac{4}{3} \end{aligned}$

Step 2: Find the gradient of the Tangent.

Taking the negative reciprocal of this, we get

$\text{Gradient of tangent } = \textcolor{blue}{-\dfrac{3}{4}}$

Step 3: Complete the rest of the equation.

Now we know the gradient, our straight-line equation must be $y = \textcolor{blue}{-\frac{3}{4}}x + c$ , where $c$ is the $y$ -intercept that we are yet to determine.

We know that this tangent passes through the point $(3,4)$ , so we can substitute these values of $x$ and $y$ into our straight-line equation and rearrange to find $c$ . We get:

$4 = \textcolor{blue}{-\dfrac{3}{4}} \times 3 + c$

$4 = -\dfrac{9}{4} + c$

$\textcolor{limegreen}{c} = 4 + \dfrac{9}{4} = \textcolor{limegreen}{\dfrac{25}{4}}$

Now we’ve found $c$ , we can express our equation of our tangent fully:

$y = \textcolor{blue}{-\dfrac{3}{4}}x + \textcolor{limegreen}{\dfrac{25}{4}}$

Circle Graphs and Tangents Example Questions

Question 1: State the equation of the circle pictured below.

[2 marks]

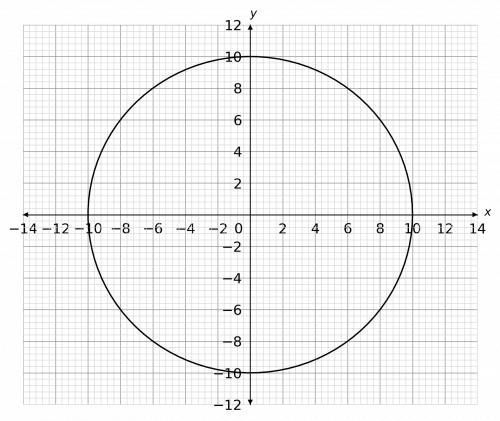
circle graphs and tangents example 1

We can see that the radius of this circle extends a distance of $10$ away from the centre at $(0,0)$ . Therefore, because $10^2 = 100$ , the equation of the circle is

$x^2 + y^2 = 100$

Save your answers with

Gold Standard Education

Question 2: Find the equation of the tangent to the circle below at the point marked with a cross.

[4 marks]

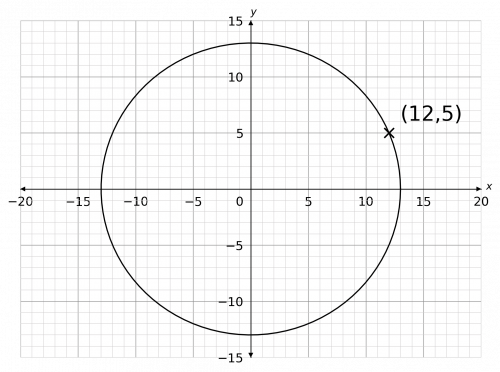
circle graphs and tangents example 2

First, we need to find the gradient of the line from the centre to $(12, 5)$ .

$\text{Gradient of radius } = \dfrac{\text{change in } y}{\text{change in }x} = \dfrac{5 - 0}{12 - 0} = \dfrac{5}{12}$

Now, by observing that this line is a radius, and that tangents are perpendicular to the radius, we can find the gradient of the tangent by taking the negative reciprocal of the answer we got above.

$\text{Gradient of tangent } = -\dfrac{12}{5}$

So, we know the straight-line equation for our tangent must be of the form

$y = -\dfrac{12}{5}x + c$ ,

where $c$ is the y-intercept which we must determine. To do this, we can substitute the values of $x = 12$ and $y = 5$ into the straight-line equation, since we know the line must pass through those coordinates. We get the following.

$5 = -\dfrac{12}{5} \times 12 + c$

$5 = -\dfrac{144}{5} + c$

$c = 5 + \dfrac{144}{5} = \dfrac{169}{5}$

So, our final answer is

$y = -\dfrac{12}{5}x + \dfrac{169}{5}$

Save your answers with

Gold Standard Education

Question 3: Find the equation of the tangent to $x^2 + y^2 = 113$ at the point $(-8,-7)$ .

[4 marks]

circle graphs and tangents example 3

First, we need to find the gradient of the line from the centre to $(-8, -7)$ .

$\text{Gradient of radius } = \dfrac{\text{change in } y}{\text{change in }x} = \dfrac{-7 - 0}{-8 - 0} = \dfrac{7}{8}$

Now, by observing that this line is a radius, and that tangents are perpendicular to the radius, we can find the gradient of the tangent by taking the negative reciprocal of the answer we got above.

$\text{Gradient of tangent } = -\dfrac{8}{7}$

So, we know the straight-line equation for our tangent must be of the form

$y = -\dfrac{8}{7}x + c$ ,

where $c$ is the y-intercept which we must determine. To do this, we can substitute the values of $x = -8$ and $y = -7$ into the straight-line equation, since we know the line must pass through those coordinates. We get the following.

$-7 =-\dfrac{8}{7} \times -8 + c$

$-7 = \dfrac{64}{7} + c$

$c=-\dfrac{113}{7}$

So, our final answer is

$y = -\dfrac{8}{7}x -\dfrac{113}{7}$

Save your answers with

Gold Standard Education