Revise
Inequalities On a Number Line
Inequalities On a Number Line Revision
Inequalities
The equations we are most familiar with are equalities, where the left hand side and the right hand side are identical or equal. It is also useful to have a way of expressing a range of values instead of a single specific value which can be achieved by using inequalities. A common way to display inequalities is using a number line.
Inequality Symbols and their Meaning
Before learning about how to display inequalities on a number line, you firstly need to know the following symbols and their definitions:
- > means “greater than”,
- \geq means “greater than or equal to”,
- < means “less than”,
- \leq means “less than or equal to”.
We call \leq and \geq inclusive inequalities, and we call < and > strict inequalities. For example x\leq 8 includes the value 8 as a possibility for x, whilst the inequality x<8 does not.
Type 1: Inequalities on a Number Line
The inequality, x\geq -5 means that x can take any value that is bigger than -5, including -5.
We can display this on a number line by drawing a filled in circle at -5 and an arrow pointing to the right hand side indicating the numbers that are greater than -5. This should look like,
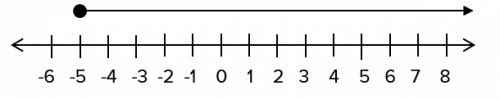
When drawing inequalities on a number line it is important to remember that you should use an closed circle, \bullet, for an inclusive inequality, e.g. x\geq-5 and use a open circle, \circ, for a strict inequality, e.g. x\lt2.
Type 2: Inequalities on a Number Line
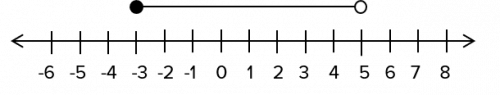
Inequalities can describe a range of values between an upper and lower limit. For example the inequality -3 \leq x \lt 5 means that x can take any value greater than or equal to -3 but also has to be less than 5.
Here, the first part of the inequality is an inclusive inequality so is drawn with a filled in circle and the second part is a strict inequality so is drawn with a empty circle.
Example: Draw -3 \leq x \lt 5 on the number line below.
Example 1
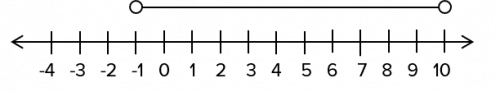
Show the inequality -1 \lt x \lt 10 on a number line.
[2 marks]
Key points when drawing the number line.
- The circles indicate the limits of the inequality (-1 and 10).
- Both are strict inequalities they should be open circles.
- A connecting line between the two circles indicates the values x can take between the two limits.
Putting it all together the number line should look like,
Example 2
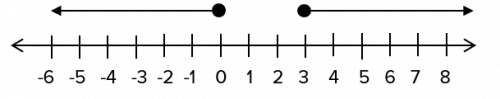
Display the inequalities x \leq 0 and x \geq 3 on a number line.
[2 marks]
This is similar to the previous example but this time,
- Both are inclusive inequalities so should be drawn with closed circles
- x can take any value less than or equal to 0 so an arrow should be drawn to the left hand side of 0
- x can take any value grater or equal to 3 so an arrow should be drawn to the right hand side of 3
Inequalities On a Number Line Example Questions
Question 1: Display the inequality -1\geq x on a number line.
[1 mark]
The inequality, -1\geq x, will require a closed circle at 3 and an arrow pointing right.
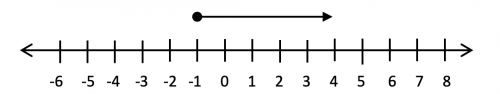
Question 2: Display the inequality x \le 4 on a number line.
[1 mark]
The inequality, x \le 4, will require an closed circle at 4 and an arrow pointing left.
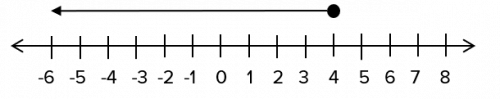
Question 3: Display the inequalities y>3 and y<-2 on the same number line.
[2 marks]
The first inequality, y>3, will require an open circle at 3 and an arrow pointing right.
The other inequality, y<-2, will require an open circle at -2 and an arrow pointing left.
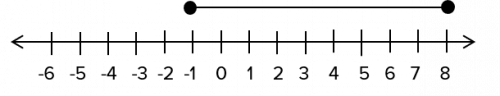
Question 4: Display the inequality -1\leq x \leq8 on a number line.
[2 marks]
The lower bound, -1\leq x, will require a closed circle at x= -1
The upper bound x \leq8, will require an closed circle at x=8
Question 5: A school offers the option of GCSE drama for year 10 and 11 students.
However, it will only go ahead if at least 8 students choose to do drama at GCSE.
Due to the classroom capacity, there has to be less than 32 students.
Write an inequality for the amount of students, s, that will be in the drama class and display this on a number line.
[3 marks]
Forming the correct inequality 8\leq s < 32 and displaying with a closed circle for representing the non-strict inequality (8) and an open circle representing the strict inequality (32).
Inequalities On a Number Line Worksheet and Example Questions
(NEW) Inequalities On a Number Line Exam Style Questions - MME
Level 4-5GCSENewOfficial MMEInequalities On a Number Line Drill Questions
Inequalities - Drill Questions
Level 4-5GCSE