Distance-Time Graphs

GCSEKS3Level 4-5AQACambridge iGCSEEdexcelEdexcel iGCSEOCRWJEC

Distance-Time Graphs Revision

Distance-Time Graphs

Distance-time graphs are a way of visually expressing a journey. With distance on the $y$ -axis and time on the $x$ -axis, a distance-time graph tells us how far someone/something has travelled and how long it took them/it to do so.

Make sure you are happy with the following topics before continuing:

Distance time graphs – Key things to remember:

1) The gradient of the line = speed

2) A flat section means no speed (stopped)

3) The steeper the graph the greater the speed

4) Negative gradient = returning to start point (coming back)

Using Distance Time Graphs

The graph below describes a journey that has several parts to it, each represented by a different straight line.

Part A: $\bf{09:00 - 11:00}$ , the person travelled $30$ km away from their starting point and that took them $2$ hours.

Part B: $\bf{11:00 - 12:00}$ , we can see that the line is flat, so the distance from their starting point did not change – they were stationary.

Part C: $\bf{12:00 - 12:30}$ , they moved a further $30$ km away from their starting point.

Part D: $\bf{12:30 - 14:00}$ , they travelled the full $60$ km back to where they began.

Calculate the speed – for each part of the journey:

$\text{Speed} (S) = \dfrac{\text{Distance}(D)}{\text{Time}(T)}$

Part A: $\bf{09:00 - 11:00}$

$\text{Speed}= \dfrac{30}{2} = 15$ km/h

Part B: $\bf{11:00 - 12:00}$

$\text{Speed}= \dfrac{0}{1} = 0$ km/h (Not moving)

Part C: $\bf{12:00 - 12:30}$

$\text{Speed}= \dfrac{30}{0.5} = 60$ km/h

Part D: $\bf{12:30 - 14:00}$

$\text{Speed}= \dfrac{60}{1.5} = 40$ km/h

From this we can see that the person travelled the fastest over part C.

Example: Bike Ride

Valentina is going for a bike ride. Below is a distance-time graph that describes her full journey.

a) How long was she stationary for?

b) What was the total distance travelled during her journey?

c) What was her average speed in kilometres per hour between $17:15$ and $17:45$ ?

[3 marks]

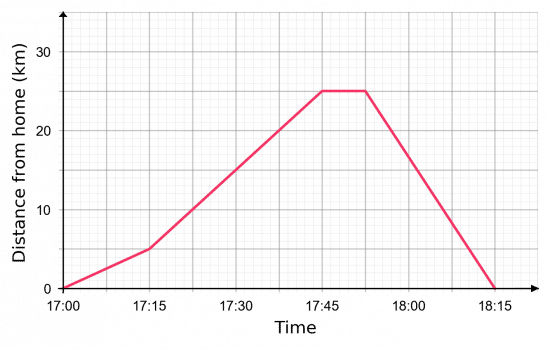
a) We can see that the graph was flat for the duration of one big square. From the axis, we can see that two big squares total $15$ minutes, therefore one big square is worth $7.5$ minutes, so she was stationary for $7.5$ minutes.

b) Valentina travelled away $25$ km away from home, stopped briefly, and then travelled $25$ km back home. Therefore, she travelled $50$ km in total.

c) We need to calculate the gradient of the graph between $17:15$ and $17:45$ . This period lasted for $30$ minutes, which is equivalent to $0.5$ hours – this is the “change in $x$ ”. During this period, she increased her distance from home from $5$ km up to $25$ km, meaning she travelled $20$ km in total – this is the “change in $y$ ”. So, we get

$\text{Gradient } = \dfrac{20}{0.5} = 40$ km/h

Distance-Time Graphs Example Questions

Question 1: From the description of Neil’s journey below, construct a distance-time graph.

Neil left home at $12:00$ and after an hour and a half of moving at a constant speed, he had travelled $44$ miles, at which point he stopped. After $3$ hours of being stopped, he drove towards home at a constant speed, and it took him $2$ hours in total to get home.

[4 marks]

So, making the words above seem more readable, we get:

$12:00 - 13:30$ , he travels from $0$ miles away to $44$ miles away;
$13:30 - 16:30$ , he stays in one place;
$16:30 - 18:30$ , he travels from $44$ miles away to $0$ miles away.

On a graph, this looks like:

Save your answers with

Gold Standard Education

Question 2: Below is a distance-time graph describing a $1500$ m race ran by Chris. Work out the maximum speed he reached during this run to 3 significant figures.

[3 marks]

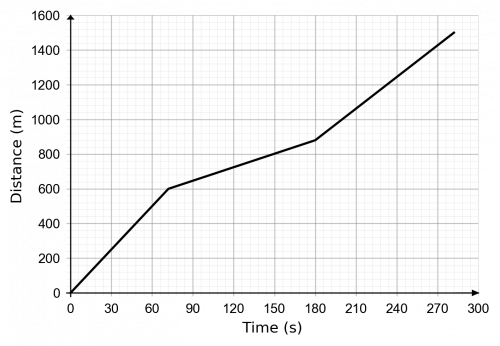
distance time graphs example 2

Looking at the graph, we can see that he runs at three different speeds during different portions of the race. The graph becomes less steep in the middle, so that won’t be his period of maximum speed, and the other two are hard to distinguish just by looking so we’ll work them both out.

Period 1:

$\text{Gradient } = \dfrac{\text{distance travelled}}{\text{time taken}} = \dfrac{600 - 0}{72 - 0} = 8.33$ m/s

Period 3:

$\text{Gradient } = \dfrac{\text{distance travelled}}{\text{time taken}} = \dfrac{1,500 - 880}{282 - 180} = 6.08$ m/s

Therefore, the fastest speed travelled by Chris during the race was $8.33$ m/s, to $3$ sf.

Save your answers with

Gold Standard Education

Question 3: A cyclist on a training ride records the distance she travels away from home. The data only shows the first $150$ minutes of the ride before her cycling computer ran out of battery.

[3 marks]

(a) How far did she ride in the first $150$ minutes?

(b) For how long was she stationary?

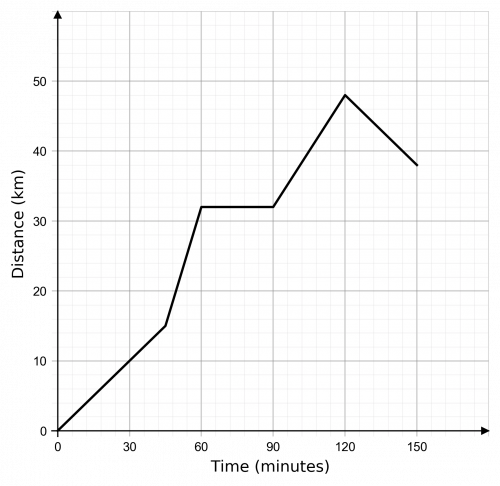
distance time graphs example 3